Spiegel Online reports today (Escher in 3D) on work of Alexander Gürten from the contest Math Creations.
It is about bulls tesselating Euclidean space. Have a look at the linked video:
http://www.spiegel.de/video/embedurl/video-1792457-640_000_fff.html
One may consider this a 3-dimensional version of M. C. Escher’s 2-dimensional patterns, similar to the one in the picture below. (We can not show Escher’s original patterns here, because they are not freely usable.)

More work of Alexander Gürten is on his website Contralex, for example the animation of the heat flow below or the project Creation of Escher Tilings.
As we know, Escher started around 1958 to produce not just Euclidean, but also hyperbolic tesselations. (The picture below is not from Escher’s but from Sylvio Levy. It is however quite close to Escher’s “Circle Limit III.)
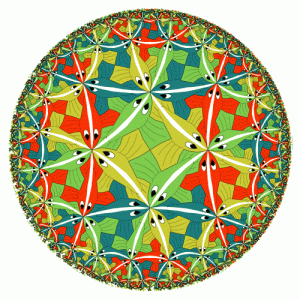
While the Euclidean plane has only 17 isomorphism classes of discrete and cocompact groups of symmetries, there is an infinitude of them for the hyperbolic plane.
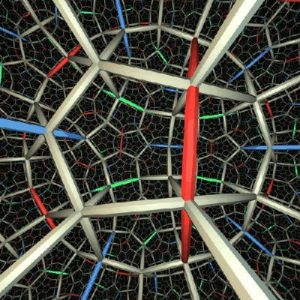
Even more possibilities are there for the discrete groups of symmetries of 3-dimensional hyperbolic space. The picture (by Charles Gunn from the movie NotKnot) shows such a 3-dimensional tesselation. It seems hard to realize this kind of tesselations in an Escher-style artistic way, but who knows?