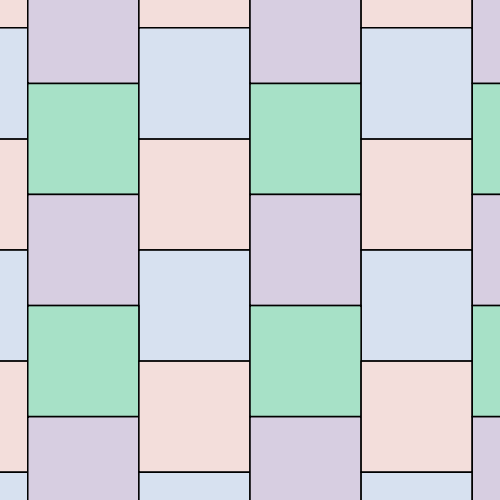
Keller’s conjecture states that in any tiling of Euclidean space by identical hypercubes there are two cubes that meet face to face.
(Consider the 2-dimensional picture on the right taken from Wikipedia. The squares share horizontal edges.)
The conjecture is completely solved by now: it is true in dimensions 7 and less, but false in higher dimensions. The last missing part was achieved a year ago (arXiv, DOI) by Brakensiek-Heule-Mackey-Narváez. There is also a Quanta article about this: link.